Draw me a picture of a Cooper pair

Note: This is a post by Brian Skinner as part of a blog exchange. He has his own blog, which I heartily recommend, called Gravity and Levity. He is currently a postdoctoral scholar at MIT in theoretical condensed matter physics.

The central, and most surprising, idea in the conventional theory of superconductivity is the notion of Cooper pairing. In a Cooper pair, two electrons with opposite momentum somehow manage to overcome their ostensibly enormous repulsive energy and bind together to make a composite bosonic particle. These composite bosons are then able to carry electric current without dissipation.

But what does a Cooper pair really look like? In this post I’m going to try to draw a picture of one, and in the process I hope to discuss a little bit of physical intuition behind how Cooper pairing is possible.

To begin with, one should acknowledge that the “electrons” that comprise Cooper pairs are not really electrons as God made them. These electrons are the quasiparticles of Fermi liquid theory, which means that they are singly-charged, half-spinned objects that are dressed in excitations of the Fermi sea around them. In particular, each “electron” that propagates through a metal carries with it a screening atmosphere made up of local perturbations in charge density. Something like this:

That distance in this picture is the Thomas-Fermi screening radius, which in metals is on the same order as the Fermi wavelength (generally Angstroms). At distances much longer than , the electron-electron interaction is screened out exponentially.

What this screening implies is that as long as the typical distance between electrons inside a Cooper pair is much longer than the Fermi wavelength (which it has to be, since there is really no concept of an electron that is smaller than the Fermi wavelength), the mutual Coulomb repulsion between electrons isn’t a problem. Electrons that are much further apart than simply don’t have any significant Coulomb interaction.

But, of course, this doesn’t explain what actually makes the electron stick together.  In the conventional theory, the “glue” between electrons is provided by the electron-phonon interaction. We typically say that electrons within a Cooper pair “exchange phonons”, and that this exchange mediates an attractive interaction. If you push a physicist to tell you what this exchange looks like in real space, you might get something like what is written in the Wikipedia article:

An electron moving through a conductor will attract nearby positive charges in the lattice. This deformation of the lattice causes another electron, with opposite spin, to move into the region of higher positive charge density. The two electrons then become correlated.

This kind of explanation might be accompanied by a picture like this one or even an animation like this one, which attempt to schematically depict how one electron distorts the lattice and creates a positively-charged well that another electron can fall into.

But I never liked these kind of pictures. Their big flaw, to my mind, is that in metals the electrons move much too fast for it to make sense. In particular, the Fermi velocity in metals is usually on the order of m/s, while the phonon velocity is a paltry m/s. So the idea that one electron can create a little potential well for another to fall into simply doesn’t make sense dynamically. By the time the potential well was created by the slow rearrangement of ions, the first electron would be long gone, and it’s hard to see any meaningful way in which the two electrons would be “paired”.

The other problem with the picture above is that it doesn’t explain why only electrons with opposite momentum can form Cooper pairs. If Cooper pairing came simply from one electron leaving behind a lattice distortion for another to couple to, then why should the pairing only work for opposite-momentum electrons?

So let me advance a different picture of a Cooper pair.

It starts by reminding you that the wavefunction for a (say) right-going electron state looks like this:

.

The probability density for the electron’s position in this state, , is uniform in space.

On the other hand, the wavefunction for a left-going electron state looks like

.

It also has a uniform probability distribution. But if you use the two states (one with momentum and the other with momentum ) to make a superposition, you can get a state whose probability distribution looks like a standing wave: .

In other words, by combining electron states with and , you can arrive at an electron state where the electron probability distribution (and therefore the electron charge density) has a static spatial pattern.

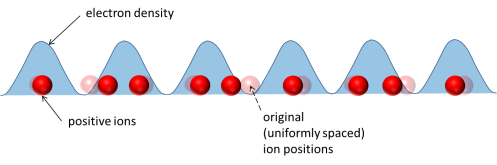
Once there is a static pattern, the positively charged ions in the crystal lattice can distort their spacing to bring themselves closer to the regions of large electron charge density. Like this:

In this way the system lowers its total Coulomb energy.  In essence, the binding of opposite-momentum electrons is a clever way of effectively bringing the fast-moving electrons to a stop, so that the slow-moving ionic lattice can accommodate itself to it.

Of course, the final piece of the picture is that the Cooper pair should have a finite size in space – the standing wave can’t actually extend on forever. This finite size is generally what we call the coherence length . Forcing the two electrons within the Cooper pair to be confined within the coherence length costs some quantum confinement energy (i.e., an increase in the electron momentum due to the uncertainty principle), and this energy cost goes like , where is the Fermi momentum. So generally speaking the length should be large enough that where is the binding energy gained from Cooper pairing.  Usually these two energy scales are on the same order, so that .

Putting it all together, my favorite picture of a Cooper pair looks something like this:

I’m certainly no expert in superconductivity, but this picture makes much more sense to me than the one in Wikipedia.

Your criticisms or refinements of it are certainly welcome.

Author’s note: Thanks to Mike Norman, who taught me this picture over lunch one day.